Differential polynomials on Non-Commutative Rings in Digital Signature scheme

secure digital signature algorithm

Authors

  • V. Jalaja Sree Vidyanikethan Engineering College, Tirupathi
  • P. Vijaykumar Vellore Institute of Technology
  • R. Elumalai Vellore Institute of Technology
  • G.S.G.N. Anjaneyulu Vellore Institute of Technology

Keywords:

Mathematics, Differential Polymonials, Non-Commutative Ring, Digital Signature, Conjugacy Problem

Abstract

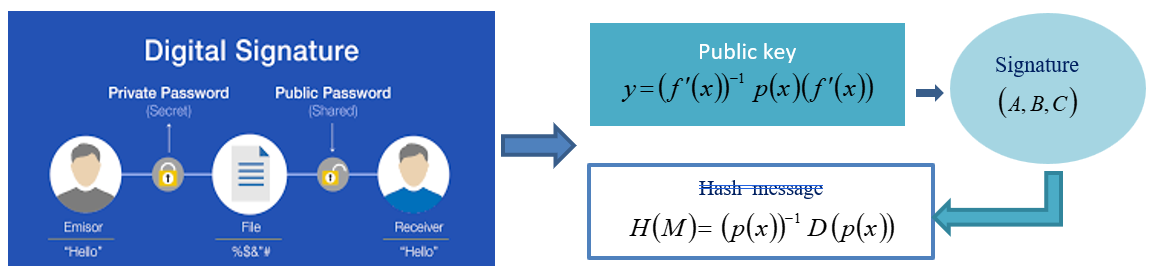
In general, digital signatures are based on difficult mathematical problems such as the discrete logarithmic problem, the Conjugacy problem, and the Integer factorization problem. In this article, authors propose a novel digital signature scheme using differential polynomials in Conjugacy problem over non-commutative structures. Initially, an algorithm on non-commutative rings in digital signatures have been generated and proved the confirmation theorem for the proposed algorithm. Finally, the signature algorithm has been evaluated for the security analysis.

URN:NBN:sciencein.jist.2023.v11.526

Author Biography

V. Jalaja, Sree Vidyanikethan Engineering College, Tirupathi

Assistant Professor of Mathematics

Downloads

Published

2023-02-26

How to Cite

Jalaja, V., Vijaykumar, P., Elumalai, R., & Anjaneyulu, G. (2023). Differential polynomials on Non-Commutative Rings in Digital Signature scheme. Journal of Integrated Science and Technology, 11(3), 526. Retrieved from https://pubs.thesciencein.org/journal/index.php/jist/article/view/a526

Issue

Vol. 11 No. 3 (2023)

Section

Computer Sciences and Mathematics

URN

https://scholar.google.com/scholar?q=urn:nbn:sciencein.jist.2023.v11.526

License

Copyright (c) 2023 V. Jalaja, P. Vijaykumar, R. Elumalai, G.S.G.N. Anjaneyulu

Creative Commons License

This work is licensed under a Creative Commons Attribution-NonCommercial-NoDerivatives 4.0 International License.

Rights and Permission