Numerical investigation of MHD nanofluid considering second-order velocity slip effect over a stretching sheet in porous media

Abstract

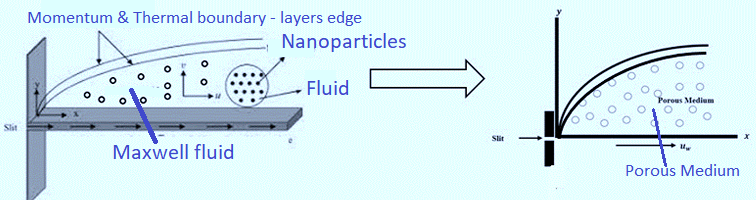
This study investigates the flow, heat, and mass transport properties of Maxwell fluid over a stretched sheet of porous media. The partial differential conditions containing second-order slip boundary conditions can be transformed into nonlinear ordinary differential conditions using proper similitude adjustments. It is possible to acquire numerical solutions for a set of transformed equations that were created from a physical model using the Runge-Kutta method with MATLAB programming. The implications of several other non-dimensional properties are also being studied. In fluid flow, many applied slip models are defined by researchers, like slip model (first-order also known as Maxwell slip model, the 1.5-order slip model, the FK model, and the second-order slip model. The wall's slip velocity boundary condition is created by adding the bulk velocity expansion to the wall collision molecules' tangential momentum transfer rate, which is then matched to the area's wall shear stress.

URN:NBN:sciencein.jist.2023.v11.478